The Trigonometric Double Angle identities or Trig Double identities actually deals with the double angle of the trigonometric functions. For instance,
- Sin2(α)
- Cos2(α)
- Tan2(α)
- Cosine2(α)
- Sec2(α)
- Cot2(α)
Double Angle identities are a special case of trig identities where the double angle is obtained by adding 2 different angles. In this article, we will cover up the different aspects of Trig Double Identities. Before Double, You must have an understanding of Trig Basic Identities.
Trig Double Identities – Trigonometric Double Angle Identities
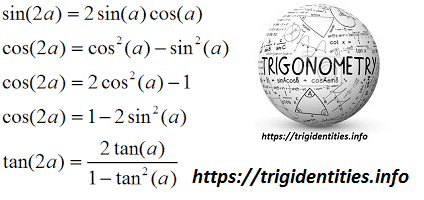
Here are some of the formulas which are expressing the trigonometric double angled identities in terms of angle x.
| Sin(2x) | = | 2sinxcosx |
| Cos(2x) | = | Cosˆ2x – Sinˆ2x |
| = | 2cosˆ2x -1 | |
| = | 1- 2sinˆx 1 | |
| Tan(2x) | = | 2tanx/1-tanˆ2x |
If you are looking for Trig Hyperbolic Identities for Trig Double Angle identities then here comes. You can also learn about Hyperbolic Trig Identities.
| Sinh (2x) | = | 2 sinh x cosh x |
| Cosh (2x) | = | 2coshˆ2 -1 |
| Tanh (2x) | = | 2 tanh x/1+tanhˆ2x |